Mastering Logarithmic Equations: A Fun Challenge for You!
Written on
Chapter 1: The Logarithm Puzzle
Have you ever engaged with puzzles involving logarithms? If you've successfully solved any, kudos to you! Here’s an intriguing challenge presented on a unique wooden board. If you can solve it in less than 30 seconds, you can officially consider yourself a logarithm expert!
Before you proceed, I suggest you pause reading, grab a pen and some paper, and attempt to solve it. Once you're ready, continue on for the solution!
Solution
The key to solving this puzzle lies in employing the change of base formula.
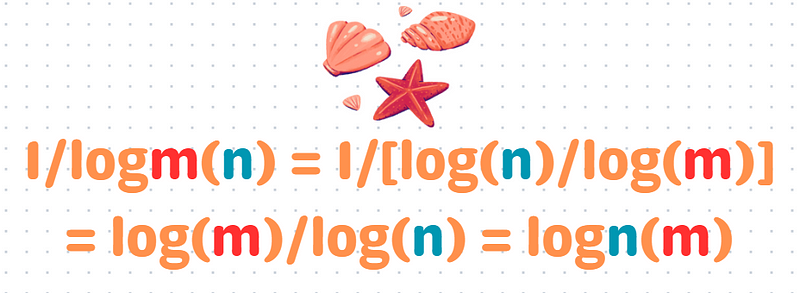
To illustrate, consider this: 1/log₂(4) equals log₄(2). By applying this concept to each term in the equation, we can eliminate the reciprocals, allowing the variables and constants to interchange their positions.
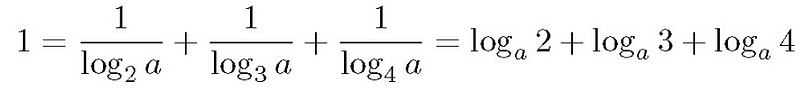
Additionally, another important property of logarithms states that log(A) + log(B) equals log(AB). This allows us to multiply the numbers 2, 3, and 4 together.
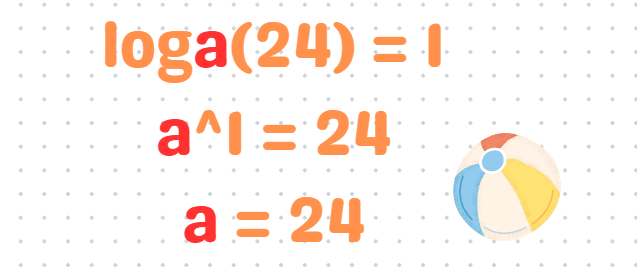
Consequently, we find that a = 24 is our solution! Isn’t that fascinating?
What were your thoughts while attempting this puzzle? I’d love to hear from you—leave a comment below!
Math Puzzles Collection
Explore the finest math puzzles available on Medium. You’ll find challenges in Algebra, Geometry, Calculus, Number Theory, and much more. Share this treasure with your friends!
The first video titled "Finding values of x in logarithmic equations" delves deeper into solving logarithmic problems. It provides a comprehensive guide and examples to enhance your understanding.
The second video, "04 - Solving Logarithmic Equations - Part 1 - Equations with Log(x)," offers a detailed walkthrough of logarithmic equations, perfect for those looking to strengthen their skills.
Thank you for taking the time to read this. If you found this article useful, don’t forget to give it a clap!

If you appreciate my work, consider supporting me with a coffee. It’s a wonderful way to encourage my writing and support my academic journey.
Happy Solving, Bella!